Sudoku 9X9

This is the regular 9X9 problem that appears in many books and newspapers. Each character must apppear exactly once in each row, column and 3X3 box.

Sudoku 9X9X

As regular 9 by 9 but must also have unique characters in each diagonal, highlighted.

Jigsaw

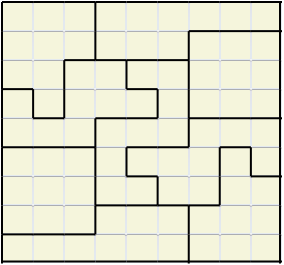
Jigsaw

As regular 9 by 9 but the normal 3X3 boxes are irregular shapes

Film

Similar to the regular 9 by 9 Sudoku but the squares have lumps in the top and bottom. The problem wraps top to bottom but not side to side so the overall geometry is a cylinder.

Fishnet

This is like a regular 9 by 9 Sudoku with holes in it.

Diamond

Here the shapes are pushed over to become diamonds. This wraps side to side but not top to bottom.

Toroid H

The normal 3X3 boxes are replaced by horizontal stripes (helices) which wrap around the problem. These stripes join the left side to the right side and the top to the bottom so that the overall geometry is a doughnut (torus).

Toroid V

The normal 3X3 boxes are replaced by vertical stripes (helices) which wrap around the problem. These stripes join the left side to the right side and the top to the bottom so that the overall geometry is a doughnut (torus).

Backslash

Must have unique characters in each row and column but the 3 by 3 boxes are replaced by backslashes. These wrap left to right and top to bottom so the overall geometry is a doughnut (torus)

People

The shapes have “lumps” on each side to become quite irregular. They look to me like little people. This problem wraps top to bottom and side to side so the overall geometry is like a doughnut (torus).

Samurai

Effectively 5 intersecting 9 by 9 Sudoku problems which must all be solved at the same time. Usually not particularly difficult problems but time consuming

3D 3X3 Cube

True 3 dimensional problem, albeit a very simple one. Each 3X3 square is one plane of a 3X3X3 cube. Consider the squares stacked on top of each other. There are 9 planes in this problem each of which is a 3X3 square which must have no repeating characters. A couple of the planes are highlighted in colour.

3D 4X4 Cube

True 3 dimensional problem. Each 4X4 square is one plane of a 4X4X4 cube. Consider the squares stacked on top of each other. There are 12 planes in this problem each of which is a 4X4 square which must have no repeating characters. Some of the planes are highlighted. There are 16 possible characters so I use a standard hexadecimal character set: 0,1,2,…,9,a,…,f.

4X4 Cube

Strictly a 2 dimensional problem since only considering the outside faces which are laid out onto a plane. There are 16 Characters which cannot repeat in any band or face. 3 of the bands are highlighted. There are 12 bands and 6 faces, each with 16 Cells. There are 16 possible characters so I use a standard hexadecimal character set: 0,1,2,…,9,a,…,f.